Lifeboat Foundation: Safeguarding Humanity
Toggle light / dark theme

Blog

Category: quantum physics – Page 115

Simulating quantum magnetism with a digital quantum computer

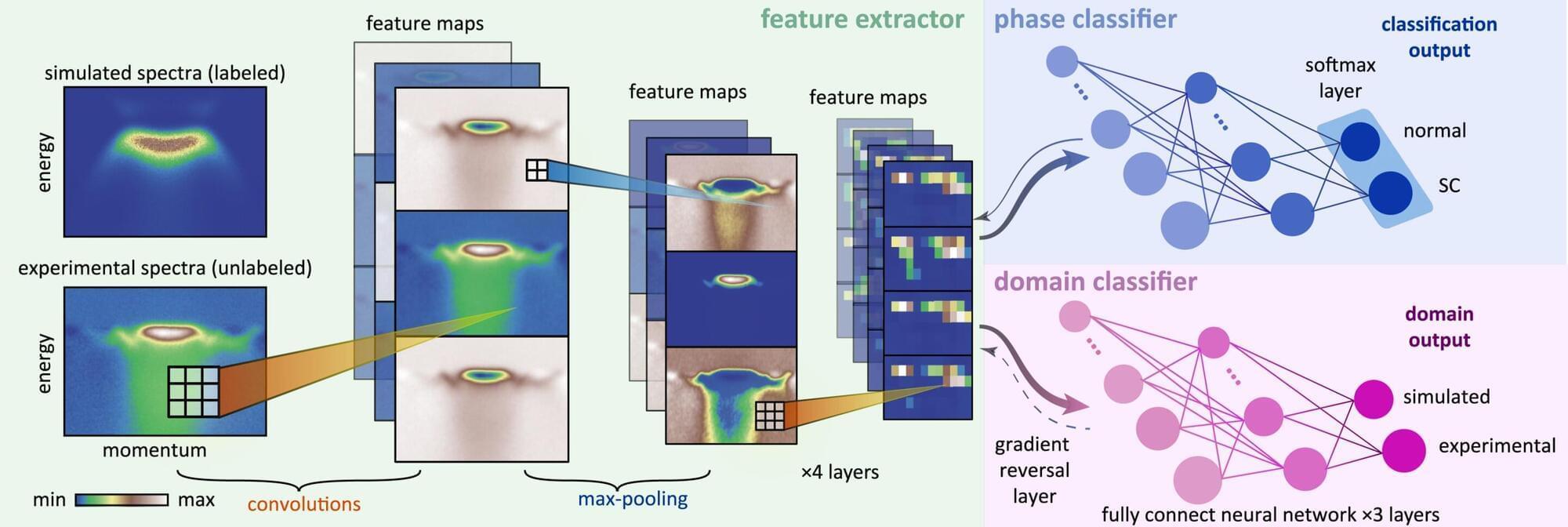
Quantum computers, which process information leveraging quantum mechanical effects, have the potential to outperform classical computers in some optimization and computational tasks. In addition, they could be used to simulate complex quantum systems that cannot be simulated using classical computers.

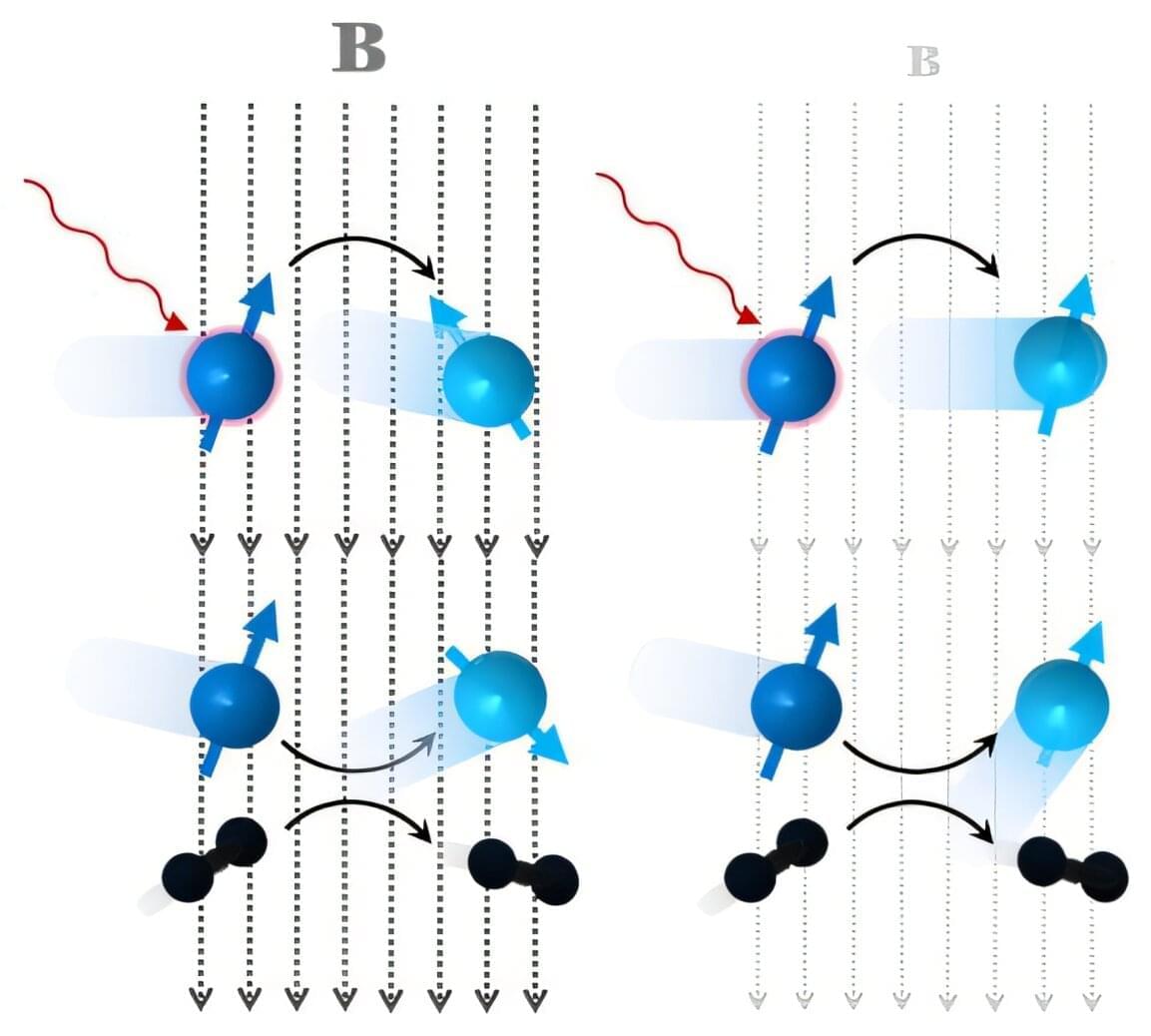
Researchers at Quantinuum and other institutes in Europe and the United States recently set out to simulate the digitized dynamics of the quantum Ising model, a framework that describes in materials, using an advanced quantum computer.

Their simulations, outlined in a paper on the arXiv preprint server, led to the observation of a transient state known as Floquet prethermalization, in which systems appear locally stable before approaching full equilibrium, in regimes that are inaccessible to classical computers.

New AI tool set to speed quest for advanced superconductors

Using artificial intelligence shortens the time to identify complex quantum phases in materials from months to minutes, finds a new study published in Newton. The breakthrough could significantly speed up research into quantum materials, particularly low-dimensional superconductors.

The study was led by theorists at Emory University and experimentalists at Yale University. Senior authors include Fang Liu and Yao Wang, assistant professors in Emory’s Department of Chemistry, and Yu He, assistant professor in Yale’s Department of Applied Physics.

The team applied to detect clear spectral signals that indicate in quantum materials—systems where electrons are strongly entangled. These materials are notoriously difficult to model with traditional physics because of their unpredictable fluctuations.

Scientists observe the first ‘quantum rain’

In the Quantum Mixtures Lab of the National Institute of Optics (Cnr-Ino), a team of researchers from Cnr, the University of Florence and the European Laboratory for Non-linear Spectroscopy (LENS) observed the phenomenon of capillary instability in an unconventional liquid: an ultradilute quantum gas. This result has important implications for the understanding and manipulation of new forms of matter.

The research, published in Physical Review Letters, also involved researchers from the Universities of Bologna, Padua, and the Basque Country (UPV/EHU).

In physics, it is known that the surface tension of a liquid, caused by intermolecular cohesive forces, tends to minimize the surface area. This mechanism is responsible for macroscopic phenomena such as the formation of raindrops or soap bubbles.

Scientists discover new way to keep quantum spins coherent longer

A new study shows that electron spins—tiny magnetic properties of atoms that can store information—can be protected from decohering (losing their quantum state) much more effectively than previously thought, simply by applying low magnetic fields.

Normally, these spins quickly lose coherence when they interact with other particles or absorb certain types of light, which limits their usefulness in technologies like or atomic clocks. But the researchers discovered that even interactions that directly relax or disrupt the spin can be significantly suppressed using weak magnetic fields.

This finding expands our understanding of how to control and opens new possibilities for developing more stable and precise quantum devices.

String Theory, Multiverse, and Divine Design — Brian Greene

Get all sides of every story and be better informed at https://ground.news/AlexOC — subscribe for 40% off unlimited access.

For early, ad-free access to videos, and to support the channel, subscribe to my Substack: https://www.alexoconnor.com.

To donate to my PayPal (thank you): http://www.paypal.me/cosmicskeptic.

VIDEO NOTES

Brian Greene is a professor of physics and mathematics at Columbia University, director of its centre for theoretical physics, and the chairman of the World Science Festival. He is best known for his work on string theory, especially in his book “The Elegant Universe”, which turns 25 this year.

LINKS.

Continue reading “String Theory, Multiverse, and Divine Design — Brian Greene” | >

When Einstein Walked with Gödel

Parul Sehgal of The New York Times stated “In these pieces, plucked from the last 20 years, Holt takes on infinity and the infinitesimal, the illusion of time, the birth of eugenics, the so-called new atheism, smartphones and distraction. It is an elegant history of recent ideas. There are a few historical correctives — he dismantles the notion that Ada Lovelace, the daughter of Lord Byron, was the first computer programmer. But he generally prefers to perch in the middle of a muddle — say, the string theory wars — and hear evidence from both sides without rushing to adjudication. The essays orbit around three chief concerns: How do we conceive of the world (metaphysics), how do we know what we know (epistemology) and how do we conduct ourselves (ethics)”. [ 6 ]

Steven Poole of The Wall Street Journal commented “…this collection of previously published essays by Jim Holt, who is one of the very best modern science writers”. [ 7 ]

[ edit ].

A new approach to probe hadronization via quantum entanglement

Recent physics studies have discovered that quarks and gluons inside protons, which are subatomic positively charged particles, exhibit maximal quantum entanglement at high energies. Entanglement is a physical phenomenon that entails correlations between distant particles that cannot be explained by classical physics theories, resulting in the state of one particle influencing that of another.

Researchers at Stony Brook University and the Brookhaven National Laboratory recently set out to better understand what this recent finding could mean for hadronization, the process by which quarks and gluons form hadrons, which are particles that can be detected experimentally. Their paper, published in Physical Review Letters, introduces a new approach to probe and study hadronization by leveraging quantum entanglement.

“Our study originated from the intriguing observation that the internal structure of protons at high energies exhibits maximal quantum entanglement,” Charles Joseph Naim, corresponding author for the paper, told Phys.org.

/* */