IBM and AMD announced plans to develop next-generation computing architectures based on the combination of quantum computers and high-performance computing, known as quantum-centric supercomputing.



Scientists at Michigan State University have discovered how to use ultrafast lasers to wiggle atoms in exotic materials, temporarily altering their electronic behavior. By combining cutting-edge microscopes with quantum simulations, they created a nanoscale switch that could revolutionize smartphones, laptops, and even future quantum computers.
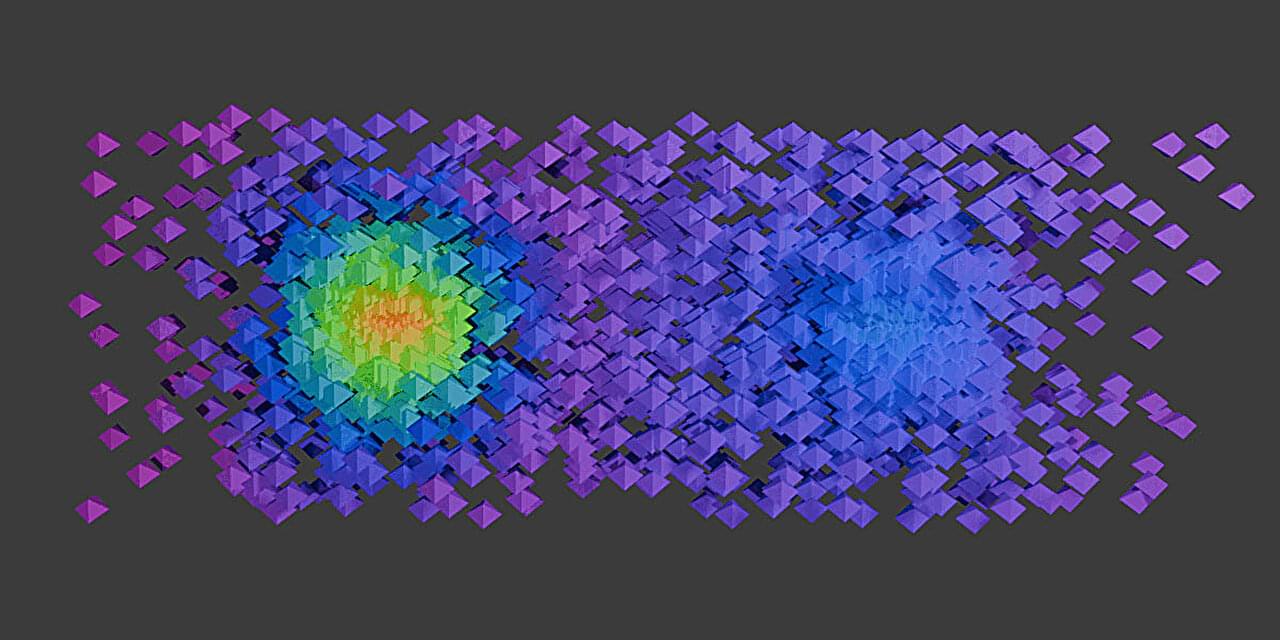
A new trick for modeling molecules with quantum accuracy takes a step toward revealing the equation at the center of a popular simulation approach, which is used in fundamental chemistry and materials science studies.
The effort to understand materials and chemical reactions eats up roughly a third of national lab supercomputer time in the U.S. The gold standard for accuracy is the quantum many-body problem, which can tell you what’s happening at the level of individual electrons. This is the key to chemical and material behaviors as electrons are responsible for chemical reactivity and bonds, electrical properties and more. However, quantum many-body calculations are so difficult that scientists can only use them to calculate atoms and molecules with a handful of electrons at a time.
Density functional theory, or DFT, is easier—the computing resources needed for its calculations scale with the number of electrons cubed, rather than rising exponentially with each new electron. Instead of following each individual electron, this theory calculates electron densities—where the electrons are most likely to be located in space. In this way, it can be used to simulate the behavior of many hundreds of atoms.

Quantum computers, computing systems that process information leveraging quantum mechanical effects, could soon outperform classical computers in various optimization and computational tasks.
To enable their reliable operation in real-world settings, however, engineers and physicists should be able to precisely control and understand the quantum states underpinning the functioning of quantum processors.
The research team led by Dapeng Yu at Shenzhen International Quantum Academy, Tongji University and other institutes in China recently introduced a new mathematical tool that could be used to characterize quantum states in quantum processors with greater accuracy.
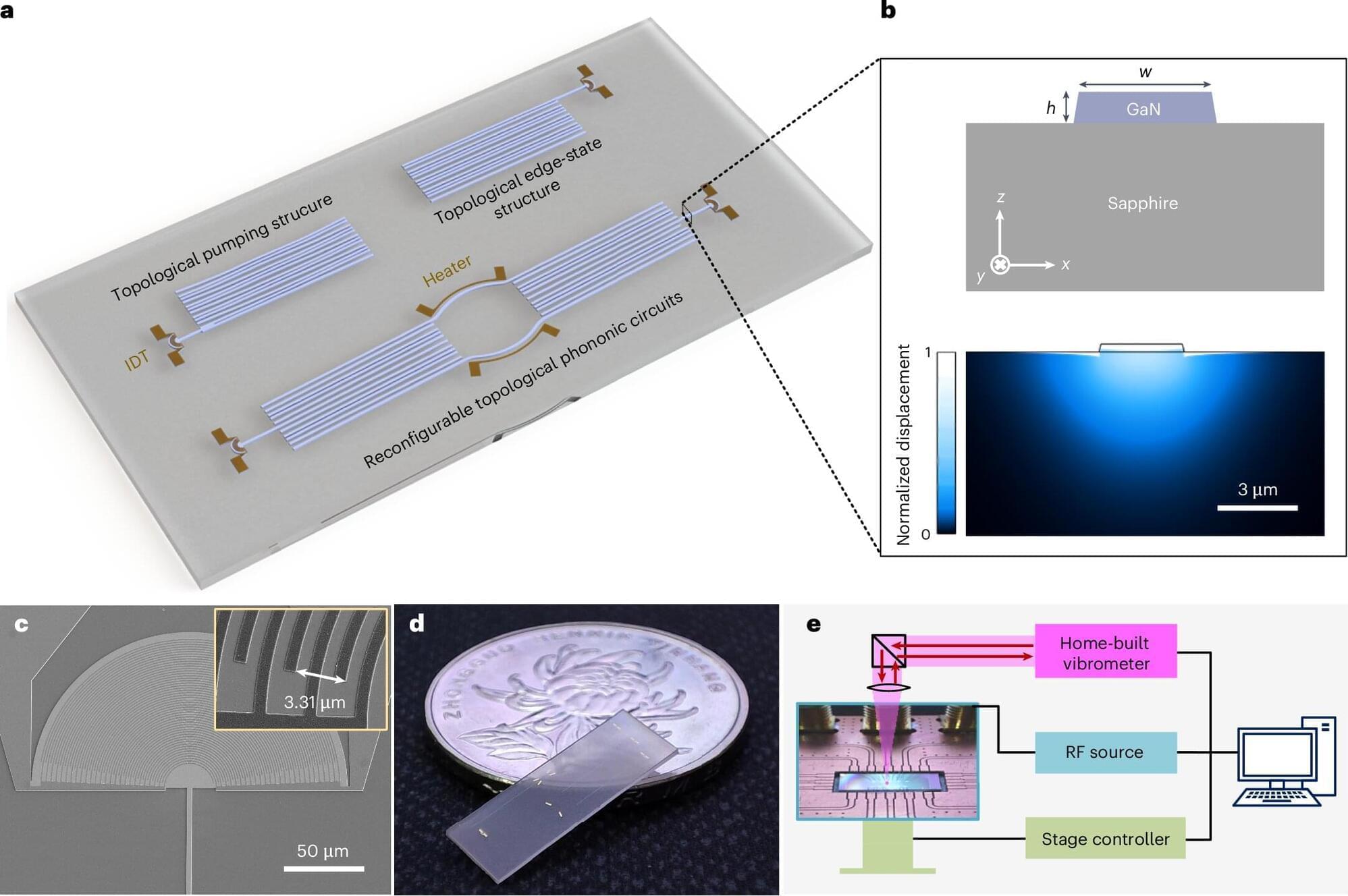
Phononic circuits are emerging devices that can manipulate sound waves (i.e., phonons) in ways that resemble how electronic circuits control the flow of electrons. Instead of relying on wires, transistors and other common electronic components, these circuits are based on waveguides, topological edge structures and other components that can guide phonons.
Phononic circuits are opening new possibilities for the development of high-speed communication systems, quantum information systems and various other technologies.
To be compatible with existing infrastructure, including current microwave communication systems, and to be used to develop highly performing quantum technologies, these circuits should ideally operate at gigahertz (GHz) frequencies. This essentially means that the sound waves they generate and manipulate oscillate billions of times per second.
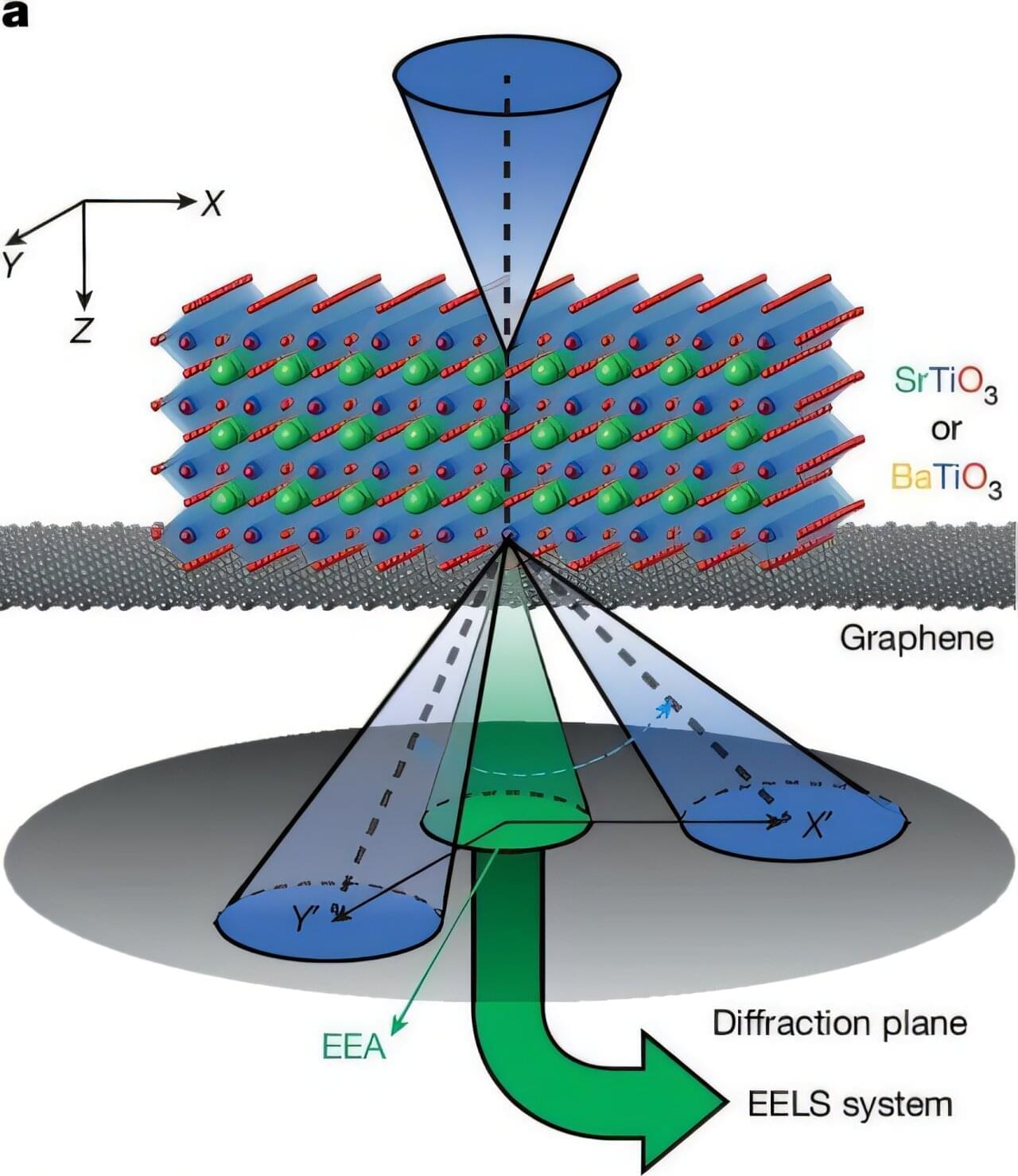
Researchers at the University of California, Irvine, together with international collaborators, have developed a new electron microscopy method that has enabled the first-ever imaging of vibrations, or phonons, in specific directions at the atomic scale.
In many crystallized materials, atoms vibrate differently along varying directions, a property known as vibrational anisotropy, which strongly influences their dielectric, thermal and even superconducting behavior. Gaining a deeper understanding of this anisotropy allows engineers to tailor materials for use in electronics, semiconductors, optics and quantum computing.
In a paper published in Nature, the UC Irvine-led team details the workings of its momentum-selective electron energy-loss spectroscopy technique and its power to unveil the fundamental lattice dynamics of functional materials.
In a collaboration showing the power of innovation and teamwork, physicists and engineers at the Department of Energy’s Oak Ridge National Laboratory developed a mobile muon detector that promises to enhance monitoring for spent nuclear fuel and help address a critical challenge for quantum computing.
Similar to neutrons, scientists use muons, fundamental subatomic particles that travel at nearly the speed of light, to allow scientists to peer deep inside matter at the atomic scale without damaging samples. However, unlike neutrons, which decay in about 10 minutes, muons decay within a couple of microseconds, posing challenges for using them to better understand the world around us.
The new detector achieves an important step toward ensuring the safety and accountability of nuclear materials and supports the development of advanced nuclear reactors that will help address the challenges of waste management. It also acts as a key step toward developing algorithms and methods to manage errors caused by cosmic radiation in qubits, the basic units of information in quantum computing. The development of the muon detector at ORNL reflects the lab’s strengths in discovery science enabled by multidisciplinary teams and powerful research tools to address national priorities.

In quantum mechanics, particles such as electrons act like waves and can even interfere with themselves—a striking and counterintuitive feature that defies our classical view of reality. We know this kind of interference happens in space, where different paths can overlap and combine, but what if we could take it further? What if we could control quantum interference in time, where electrons created at different moments interfere?
In a new study published in Physical Review Letters, a team of researchers developed a novel technique—chirped laser-assisted dynamic interference—to manipulate temporal quantum interference during photoionization.
By using extreme-ultraviolet pulses with time-varying central frequency, in combination with intense infrared laser fields, they guided electron motion with unprecedented precision.
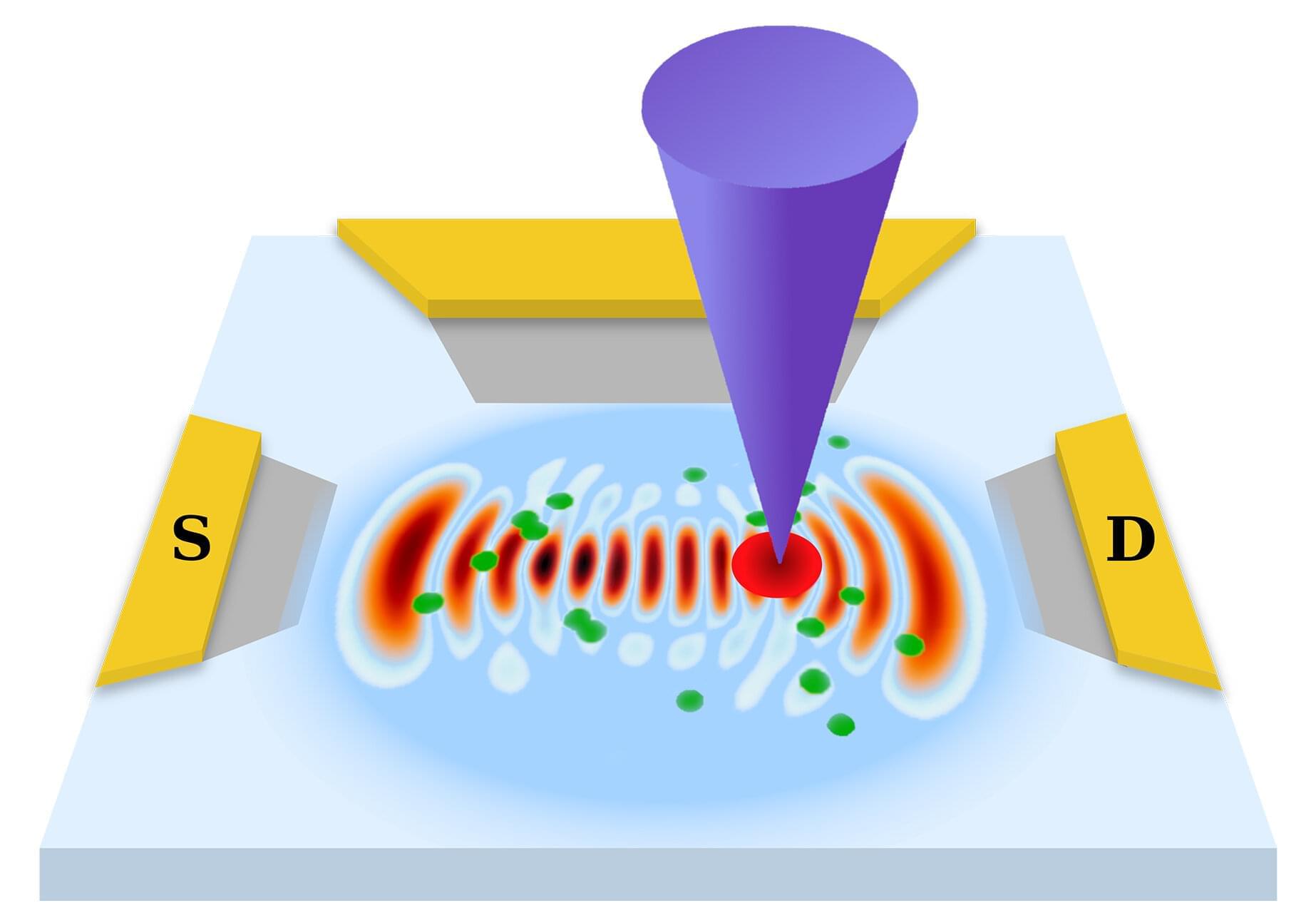
Quantum physics often reveals phenomena that defy common sense. A new theory of quantum scarring deepens our understanding of the connection between the quantum world and classical mechanics, sheds light on earlier findings and marks a step forward toward future technological applications.
Quantum mechanics describes the behavior of matter and energy at microscopic scales, where randomness seems to prevail. Yet even within seemingly chaotic systems, hidden order may lie beneath the surface. Quantum scars are one such example: they are regions where electrons prefer to travel along specific pathways instead of spreading out uniformly.
Researchers at Tampere University and Harvard University previously demonstrated in their article published in “Quantum Lissajous Scars” that quantum scars can form strong, distinctive patterns in nanostructures, and that their shapes can even be controlled. Now, the Quantum Control and Dynamics research group at Tampere University’s Physics Unit is taking these findings further. In their new article, the researchers report that quantum scars significantly enhance electron transport in open quantum dots connected to electrodes. The work is published in the journal Physical Review B.

Kyoto, Japan — The concept of quantum entanglement is emblematic of the gap between classical and quantum physics. Referring to a situation in which it is impossible to describe the physics of each photon separately, this key characteristic of quantum mechanics defies the classical expectation that each particle should have a reality of its own, which gravely concerned Einstein. Understanding the potential of this concept is essential for the realization of powerful new quantum technologies.