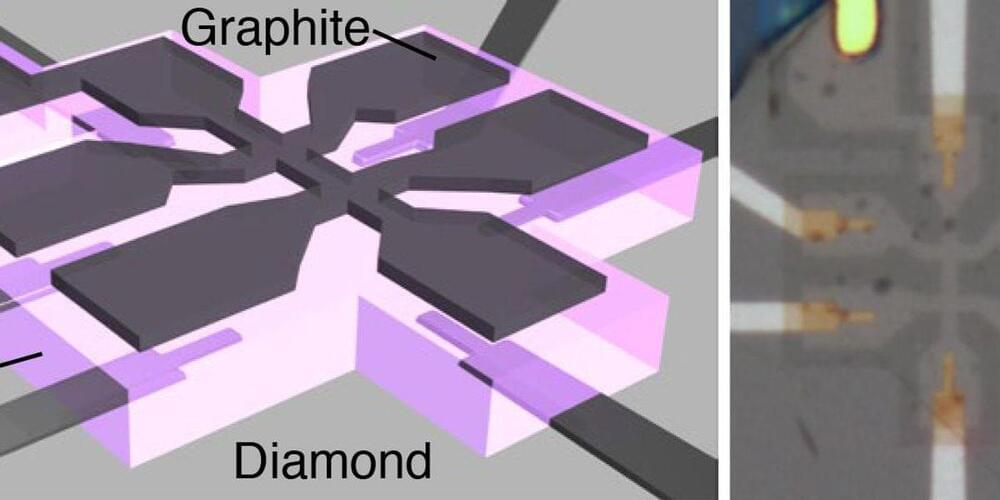
Researchers in Japan have developed a diamond FET with high hole mobility.
In the 1970s, Stephen Hawking found that an isolated black hole would emit radiation but only when considered quantum mechanics. This is known as black hole evaporation because the black hole shrinks. However, this led to the black hole information paradox.
If the black hole evaporates entirely, physical information would permanently disappear in a black hole. However, this violates a core precept of quantum physics: the information cannot vanish from the Universe.
A new study by an international quartet of physicists suggests that black holes are more complex than originally understood. They have a gravitational field that, at the quantum level, encodes information about how they were formed.