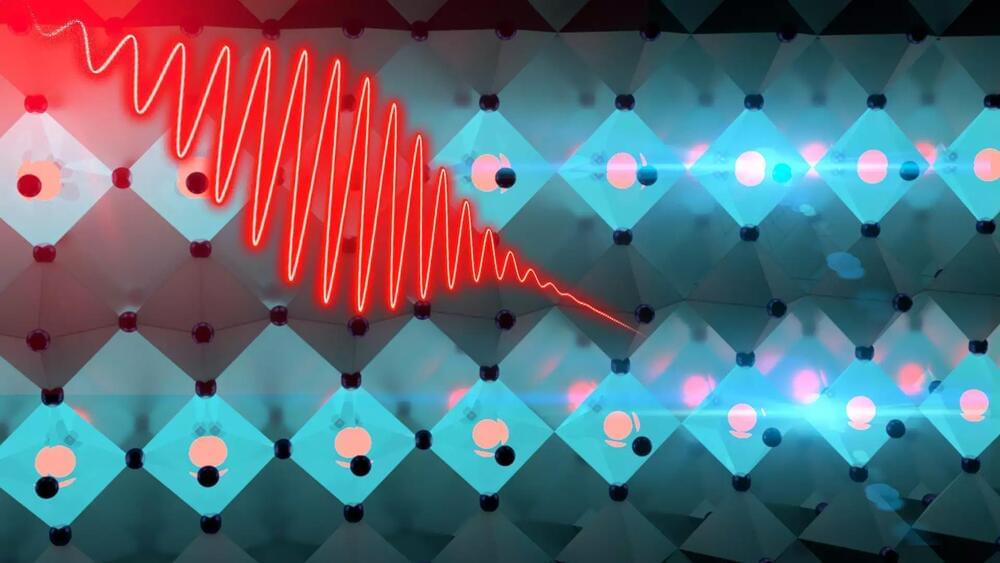
Scientists from the Max Planck Institute for Polymer Research, Paderborn University, and the University of Konstanz have succeeded in achieving a rare quantum state. They are the first to have demonstrated Wannier-Stark localization in a polycrystalline substance. Predicted around 80 years ago, the effect has only recently been proven — in a monocrystal. Until now, researchers assumed this localization to be possible only in such monocrystalline substances which are very complicated to produce. The new findings represent a breakthrough in the field of physics and could in future give rise to new optical modulators, for example, that can be used in information technologies based on light, among other things. The physicists have published their findings in the well-respected technical journal, Nature Communications.
Stronger and faster than lightning
The atoms of a crystal are arranged in a three-dimensional grid, held together by chemical bonds. These bonds can, however, be dissolved by very strong electric fields which displace atoms, even going so far as to introduce so much energy into the crystal that it is destroyed. This is what happens when lightning strikes and materials liquefy, vaporize or combust, for example. To demonstrate Wannier-Stark localization, the scientists’ experiments involved setting up electric fields of several million volts per centimeter, much stronger than the fields involved in lightning strikes. During this process, the electronic system of a solid — in this case, a polycrystal — is forced far from a state of equilibrium for a very short time. Wannier-Stark localization involves virtually shutting down some of the chemical bonds temporarily. This state can only be maintained for less than a picosecond — one millionth of one millionth of a second — without destroying the substance.