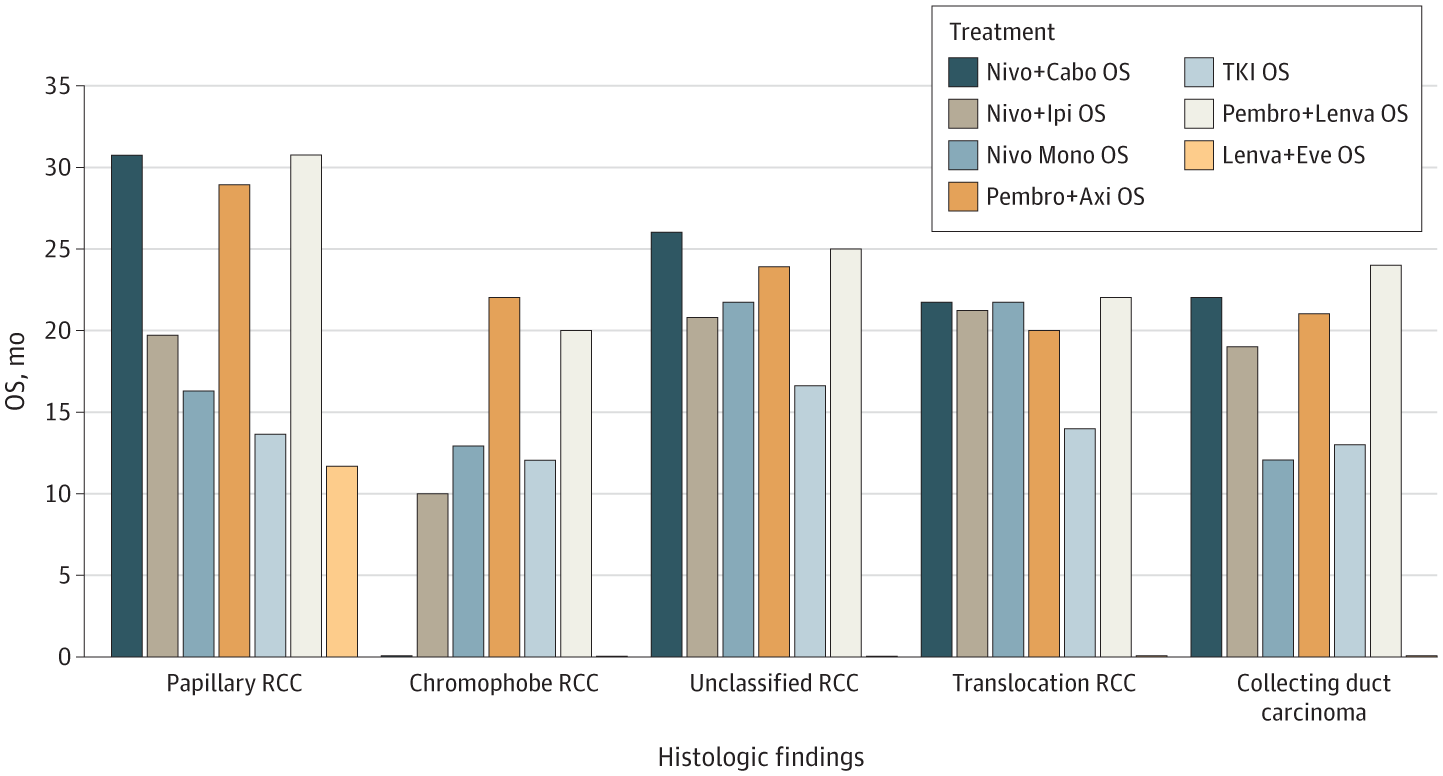
Join us on Patreon! https://www.patreon.com/MichaelLustgartenPhDDiscount Links/Affiliates: Blood testing (where I get the majority of my labs): https://www.u…
Get the latest international news and world events from around the world.

“We’re Finally Fast Enough”: Nuclear Propulsion and Solar Sails Could Blast a Spacecraft to Sedna in Just 7 Years
IN A NUTSHELL 🚀 Scientists are developing two experimental propulsion methods—nuclear fusion and solar sails—to reach Sedna. 🌌 Sedna, named after the Inuit goddess of the ocean, offers a rare chance to explore the outer solar system. 🔬 Exploring Sedna could unlock insights into the early solar system and the formation of celestial bodies. 🌍


New system helps robotic arm navigate using sound instead of vision
A new sensing system called SonicBoom could help agricultural robots navigate cluttered environments where visual sensors struggle.
Developed by researchers at Carnegie Mellon University, SonicBoom uses tiny contact microphones to sense sound and localize objects that a robotic arm touches.
Interestingly, these robots could help farmers harvest crops even in increasingly challenging conditions, such as rising temperatures.

Radio signal from the very early universe offers clues about the first stars
Understanding how the universe transitioned from darkness to light with the formation of the first stars and galaxies is a key turning point in the universe’s development, known as the Cosmic Dawn. However, even with the most powerful telescopes, we can’t directly observe these earliest stars, so determining their properties is one of the biggest challenges in astronomy.
Now, an international group of astronomers led by the University of Cambridge has shown that we will be able to learn about the masses of the earliest stars by studying a specific radio signal—created by hydrogen atoms filling the gaps between star-forming regions—originating just a hundred million years after the Big Bang.
By studying how the first stars and their remnants affected this signal, called the 21-centimeter signal, the researchers have shown that future radio telescopes will help us understand the very early universe, and how it transformed from a nearly homogeneous mass of mostly hydrogen to the incredible complexity we see today. Their results are reported in the journal Nature Astronomy.
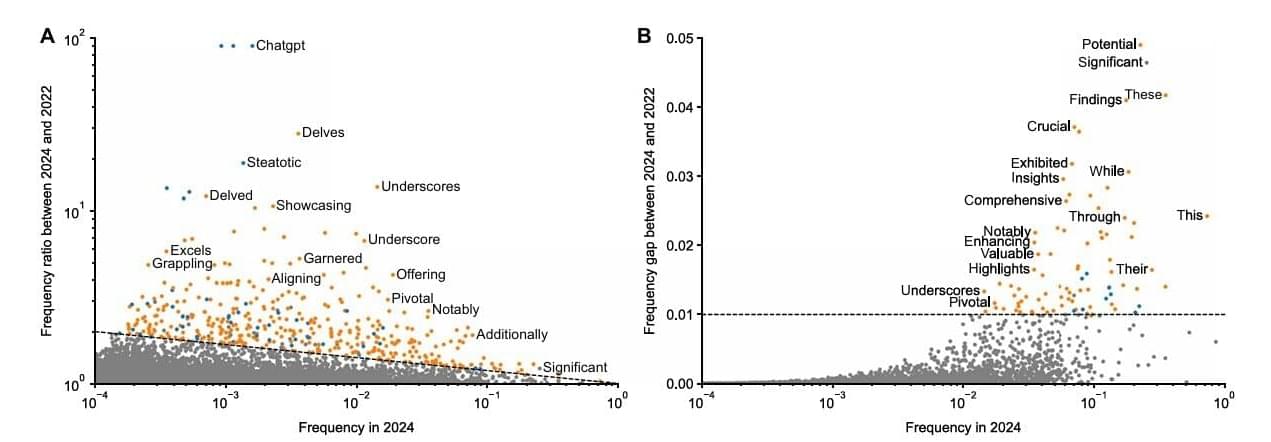
Massive study detects AI fingerprints in millions of scientific papers
Chances are that you have unknowingly encountered compelling online content that was created, either wholly or in part, by some version of a Large Language Model (LLM). As these AI resources, like ChatGPT and Google Gemini, become more proficient at generating near-human-quality writing, it has become more difficult to distinguish between purely human writing from content that was either modified or entirely generated by LLMs.
This spike in questionable authorship has raised concerns in the academic community that AI-generated content has been quietly creeping into peer-reviewed publications.
To shed light on just how widespread LLM content is in academic writing, a team of U.S. and German researchers analyzed more than 15 million biomedical abstracts on PubMed to determine if LLMs have had a detectable impact on specific word choices in journal articles.

Data Science and Machine Learning: Mathematical and
D.P. Kroese, Z.I. Botev, T. Taimre, R. Vaisman. Data Science and Machine Learning: Mathematical and Statistical Methods, Chapman and Hall/CRC, Boca Raton, 2019.
The purpose of this book is to provide an accessible, yet comprehensive textbook intended for students interested in gaining a better understanding of the mathematics and statistics that underpin the rich variety of ideas and machine learning algorithms in data science.