A trio of animal physiologists at the University of Tübingen, in Germany, has found that at least one species of crow has the ability to recognize geometric regularity. In their study published in the journal Science Advances, Philipp Schmidbauer, Madita Hahn and Andreas Nieder conducted several experiments that involved testing crows on their ability to recognize geometric shapes.
Recognizing regularity in geometric shapes means being able to pick out one shape that is different from others in a group—picking out a plastic star, for example, when it is placed among several plastic moons. Testing for the ability to recognize geometric regularity has been done with many animals, including chimps and bonobos. Until now, this ability has never been observed in any creature except for humans.
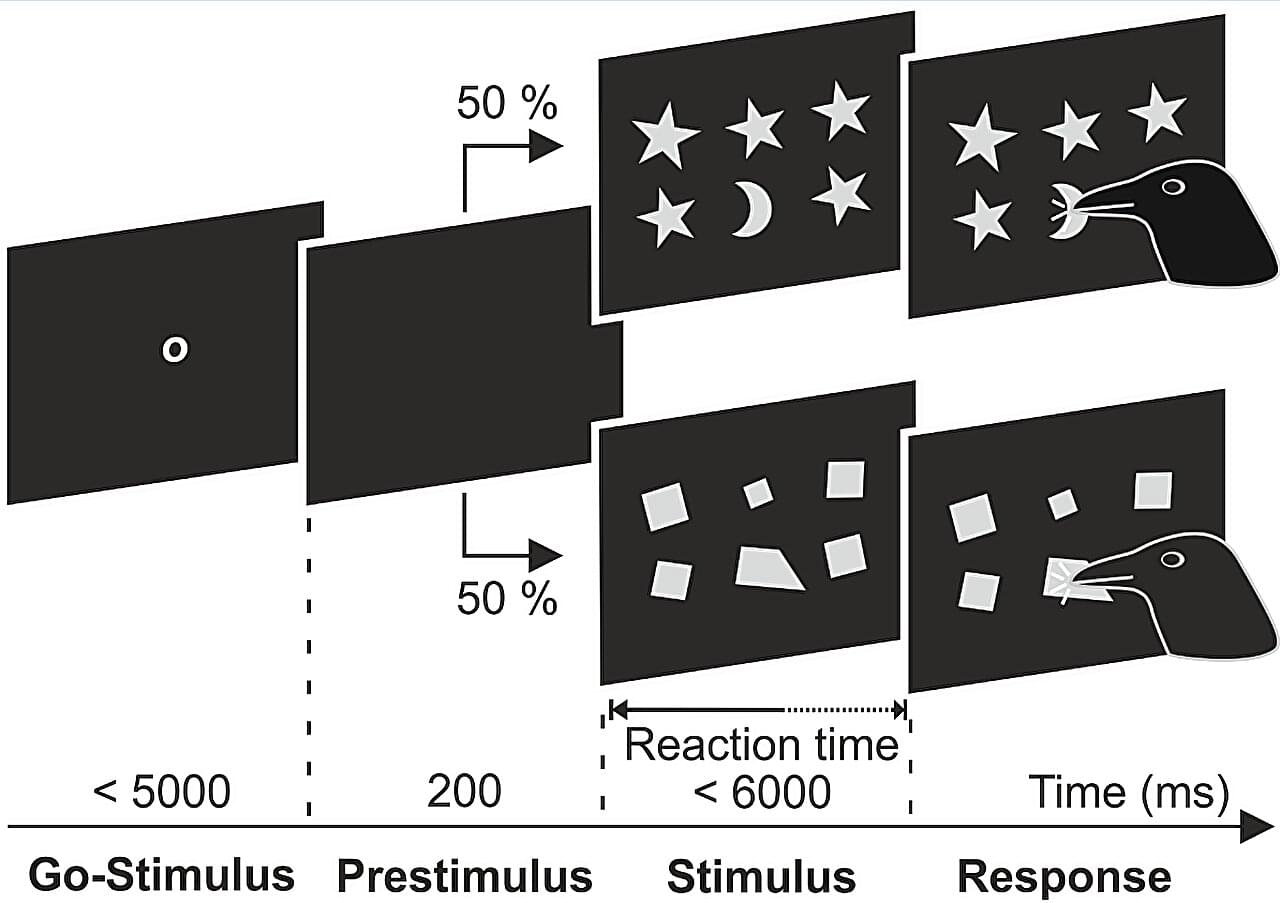
Because of that, the team started with a bit of skepticism when they began testing carrion crows. In their work, the testing was done using computer screens—the birds were asked to peck the outlier in a group; if they chose correctly, they got a food treat. The team chose to test carrion crows because prior experiments have shown them to have exceptional intelligence and mathematical capabilities.