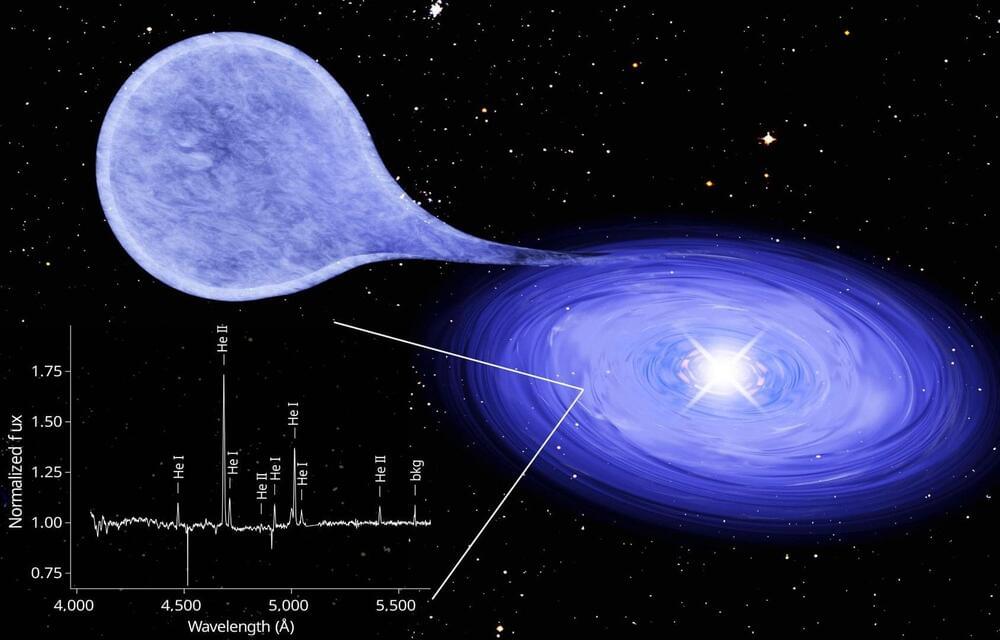
A white dwarf star can explode as a supernova when its mass exceeds the limit of about 1.4 solar masses. A team led by the Max Planck Institute for Extraterrestrial Physics (MPE) in Garching and involving the University of Bonn has now found a binary star system in which matter flows onto the white dwarf from its companion.
The system was found due to bright, so-called super-soft X-rays, which originate in the nuclear fusion of the overflowed gas near the surface of the white dwarf. The unusual thing about this source is that it is helium and not hydrogen that overflows and burns. The measured luminosity suggests that the mass of the white dwarf is growing more slowly than previously thought possible, which may help to understand the number of supernovae caused by exploding white dwarfs. The results have been published in the journal Nature.
Exploding white dwarfs are not only considered the main source of iron in the universe, they are also an important tool for cosmology. As so-called Type Ia supernovae (SN Ia), they all become roughly equally bright, allowing astrophysics a precise determination of the distance of their host galaxies.