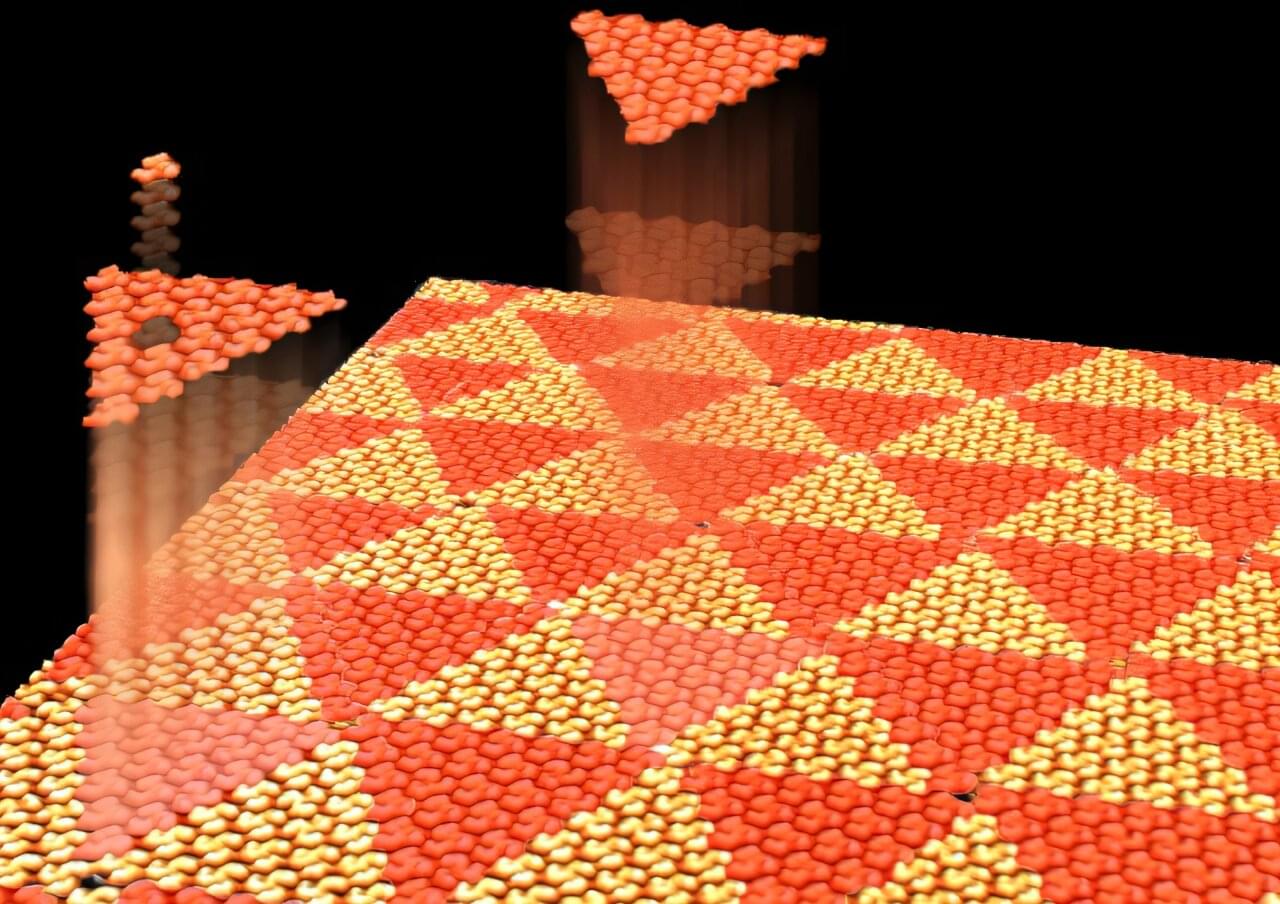
Standing at the intersection between mathematics and the tiler’s trade is the so-called einstein problem. Despite its name, this mathematical question has nothing to do with the Nobel Prize winner Albert Einstein. It asks: Can you seamlessly tile an endless surface with a single shape (an “einstein”) in such a way that the resulting pattern is never repeated? Such a “proto-tile” was first discovered in 2022 by the English amateur mathematician David Smith.

Empa researcher Karl-Heinz Ernst is neither a mathematician nor a tiler. As a chemist, he researches the crystallization of molecules on metal surfaces. He never expected to deal with the einstein problem in his professional life—until his doctoral student Jan Voigt approached him with the unusual results of an experiment.

When a certain molecule crystallized on a silver surface, instead of the expected regular structure, irregular patterns were formed that never seemed to repeat themselves. Even more surprising: Each time he repeated the experiment, different aperiodic patterns emerged.