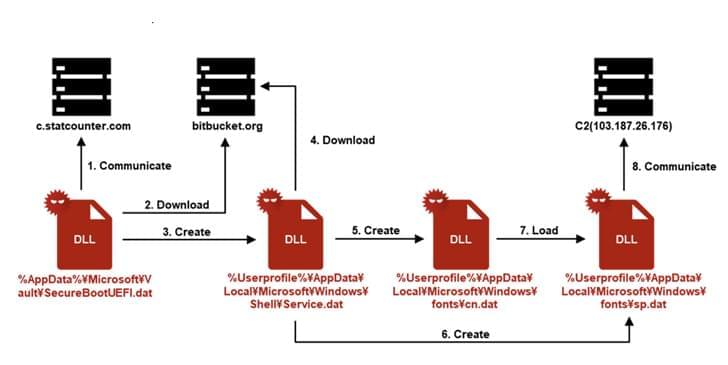
T-Mobile thwarts cyber intrusion from wireline provider’s network, ensuring no data breach or service disruption.
APT-C-60 exploits WPS Office flaw to deliver SpyGlace malware via phishing, targeting Japan with advanced techniques.
A unique Einstein zig-zag gravitational lens refines Hubble constant measurements and probes dark energy, solving key cosmological puzzles.
Layer codes Communications
Posted in futurism
Quantum error correcting codes are inefficient when implemented on platforms with local connectivity in two dimensions, which motivates platforms with 3D connectivity and codes applicable in these conditions. Here, the authors show how to transform any CSS stabilizer code into a 3D topological code, leading to codes with optimal asymptotic scaling in 3D.
Hundreds of LLM-powered AI agents spontaneously made friends, invented jobs, and spread religion.
At the center of all leading particle physics labs are the particle accelerators that make the research possible. But just why are particle accelerators necessary? What do they do? In this video, Fermilab’s Dr. Don explains it all.
Quark gluon plasma: • quark gluon plasma.
Fermilab physics 101:
https://www.fnal.gov/pub/science/part…
Fermilab home page:
https://fnal.gov
🩸📉🧬
Research found that younger age at type 2 diabetes diagnosis is associated with higher risks of cardiovascular and all-cause mortality, emphasizing the need for early prevention and personalized management strategies.
Such a cool idea: a 1 km diameter exoplanet telescope lens built from self-assembling subunits could image other worlds with incredible detail.
With the recent SpaceX Starship orbital flight tests, it is time to commit to building the largest physically possible space telescope. Such a telescope would peer deeper into the universe than any before it, answering fundamental questions: are we alone? What do Earth-like exoplanets around other stars look like? How did we get here? What weird stuff awaits discovery? Where is the limit on human ambition to know what is in our universe? The Monster Scope answers these questions. Monster, because of its enormous scale, grotesque in its ambition. Monster, from the Latin root meaning a revealed thing. And monster, because through it we may be able to study not just the rocks and land masses but possibly lifeforms, both monstrous and marvelous, on distant planets.
When we look up into the night sky, we see thousands of stars. Most of them, visible to our weak and poorly-evolved eyes, are either exceptionally close or exceptionally bright. Along with the starlight that passes each moment through our corneas onto our retinas, its brother and sister photons splash uselessly onto the skin of our face, the ground around our feet, and the rest of the entire planet.
A telescope gathers this wasted light and corrals it into exquisitely sensitive instruments, extracting more of the ambient information that otherwise flows unseen and unstudied around us. The larger the telescope, the smaller and fainter the things it can see. Our pupils are but a few millimeters across, while the James Webb Space Telescope (JWST), one thousand times larger, can see objects millions of times fainter. For telescopes, a simple rule applies: the bigger, the better!
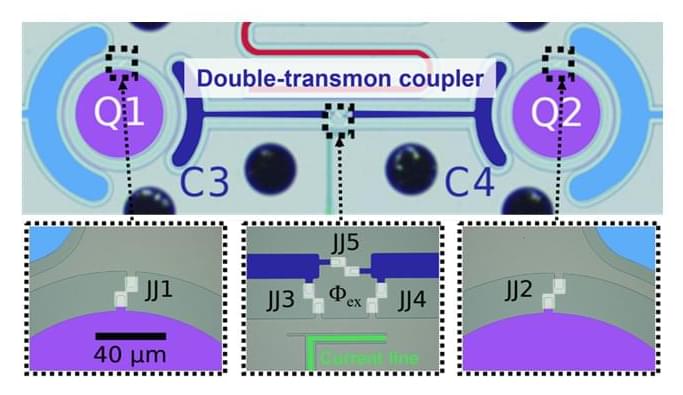
PRESS RELEASE —-Toshiba Corporation (Toshiba) has confirmed a technology that they claimed promises to advance progress toward the development of higher-performance quantum computers through an investigation of a potential advance in quantum computing. Experiments conducted by a joint research group from Toshiba and RIKEN, one of Japan’s largest comprehensive research institutions, have successfully realized a Double-Transmon Coupler, a solution for superconducting quantum computers initially proposed by Toshiba. The researchers achieved a world-class fidelity of 99.90% for a two-qubit gate, which is at the heart of quantum computation. Fidelity is a standard performance indicator for quantum gates, quantifying how close an operation is to the ideal in a range from 0% to 100%, with higher percentages indicating greater accuracy in the quantum gate’s operation.
Originally proposed by Toshiba in a paper from September 2022, the Double-Transmon Coupler is a tunable coupler that holds the key to improving the performance of superconducting quantum computers. In successful experimental realization, Toshiba and RIKEN have confirmed its theoretical superiority over conventional tunable couplers in suppressing the long-standing problem of unnecessary residual coupling and enabling high-speed, high-fidelity two-qubit gates.
To improve the performance of two-qubit gates, the coherence time, the period for which the quantum superposition state can be maintained — critical in quantum computers — must be extended. Gates must also be executed quickly and the strength of residual coupling must be suppressed to reduce the errors it causes. The Toshiba-RIKEN team achieved a world-class coherence time for the transmon qubit, a short gate time of 48 ns, and reduced the residual coupling strength to as low as 6 kHz, thereby achieving a fidelity of 99.90%.
A research team from the Shanghai Institute of Nutrition and Health (SINH) of the Chinese Academy of Sciences has revealed that aging specifically impairs the generation of CD8+ tissue resident memory T cells (TRM) and thus compromises the antitumor defensive activity of aged CD8+ T cells. The study is published in Nature Aging.
With the aging process, the risk of developing cancer significantly increases. In recent years, it has been reported that immune aging has an important impact on tumor development. Immune aging is a degenerative change in the immune system that occurs with aging, leading to a decline in immune response and ultimately triggering diseases including tumors.
Within the immune system, CD8+ T cells are the main defensive adaptive immune cells protecting against tumor cells. However, the mechanism by which aging impairs the antitumor response of CD8+ T cells was not previously understood.