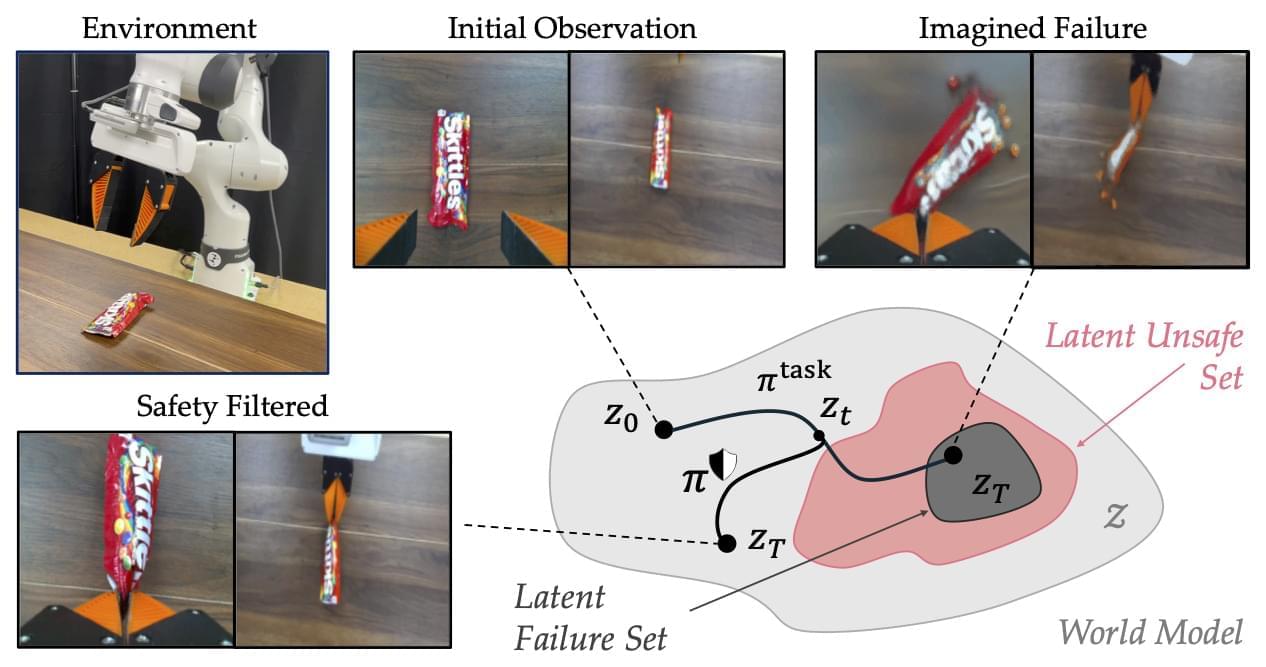
Hamilton-Jacobi (HJ) reachability is a rigorous mathematical framework that enables robots to simultaneously detect unsafe states and generate actions that prevent future failures. While in theory, HJ reachability can synthesize safe controllers for nonlinear systems and nonconvex constraints.

In practice, it has been limited to hand-engineered collision

Avoidance constraints modeled via low-dimensional state-space representations and first-principles dynamics. In this work, our goal is to generalize safe robot controllers to prevent failures that are hard—if not impossible—to write down by hand, but can be intuitively identified from high-dimensional observations: