Vorticity, a measure of the local rotation or swirling motion in a fluid, has long been studied by physicists and mathematicians. The dynamics of vorticity is governed by the famed Navier-Stokes equations, which tell us that vorticity is produced by the passage of fluid past walls. Moreover, due to their internal resistance to being sheared, viscous fluids will diffuse the vorticity within them and so any persistent swirling motions will require a constant resupply of vorticity.
Physicists at the University of Chicago and applied mathematicians at the Flatiron Institute recently carried out a study exploring the behavior of viscous fluids in which tiny rotating particles were suspended, acting as local, mobile sources of vorticity. Their paper, published in Nature Physics, outlines fluid behaviors that were never observed before, characterized by self-propulsion, flocking and the emergence of chiral active phases.
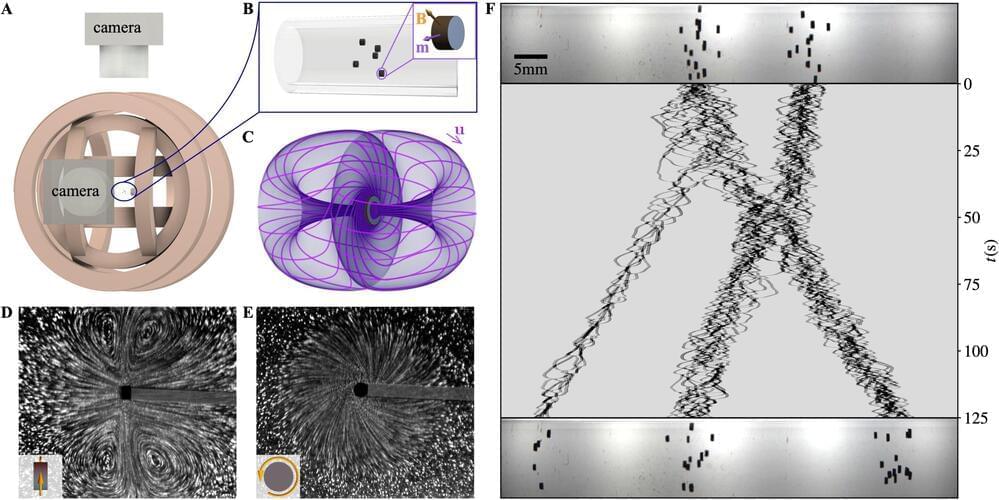
“This experiment was a confluence of three curiosities,” William T.M. Irvine, a corresponding author of the paper, told Phys.org. “We had been studying and engineering parity-breaking meta-fluids with fundamentally new properties in 2D and were interested to see how a three-dimensional analog would behave.